HW for 4/10/2016

Here is the link for Homework

HW for 4/10/2016

HW due on 03/27/2015

Here is the next week's  Home Work

What am I

1.     I can be called a rectangle, a quadrilateral, a rhombus, and a parallelogram. All of my sides are equal and I have four right angles. What am I?

2.     I am a quadrilateral. Two of my sides are parallel and two are not. What am I?

3.     I am a quadrilateral with two pairs of parallel sides. My opposite sides are equal in length and my opposite angles are equal. What am I?

4.     I am a 4 sided figure with no parallel sides. What am I?

5.     I am both a quadrilateral and a parallelogram. All four of my sides are the same length. My opposite angles are equal. What am I?

PERIMETER

   1. Find the perimeter of the triangle with the sides of 8 cm, 5 cm and 9 cm long. 

   2. Find the perimeter of the triangle, if the first side is of 8 cm long, the second side is in 4 cm     longer, and the third side measure is half of the sum of that of the first and the second sides. 

   3.Find the missed side of the triangle, if the first side is 7 cm long, the second side is 2 cm shorter and the perimeter of the triangle is 16 cm. 

   4.In a triangle, the second side is in 7 cm longer than the first one and the third side is in 4 cm shorter than the second one. Find the measures of the triangle sides, if the perimeter of the triangle is 40 cm. 

   5.Find the measures of the triangle sides, if the second side is in 6 cm shorter than the first one, the third side measure is half of the sum of the measures of the first and the second sides, and the perimeter of the triangle is 30 cm.

PERIMETER

PERIMETER

The perimeter of a polygon is equal to the sum of the length of its sides.

PERIMETER OF A TRIANGLE

Equilateral Triangle

Isosceles Triangle

Scalene Triangle

Square

Rectangle

Rhombus

Rhomboid

P = 2 · (a + b)

Regular Polygon

Circle

Polygons

POLYGON

A polygon is a closed shape with three or more straight sides and angles.

CHARACTERISTICS OF A POLYGON

• Closed figure
• Has at least three sides
• At least three angles
• No curved lines
• No intersecting lines

TYPES OF POLYGONS

Convex Polygon

All of its angles are less than 180°.

All of the diagonals are internal.

Concave Polygon

At least one angle measures more than 180 degree

At least one of the diagonals is outside the shape of the polygon.

Equilateral Polygon

All sides are equal.

Equiangular Polygons

All angles are equal.

Regular Polygon

They have equal angles and sides

Irregular Polygon 

They do not have equal angles and sides.

Types of Polygons based on Number of Sides

# of Sides	Name
3	Triangle
4	Quadrilateral
5	Pentagon
6	Hexagon
7	Heptagon
8	Octagon
9	Nonagon
10	Decagon

Home Work 2/27/2016

Here is the next week's  Home Work and Geometric Sense

LCM AND GCF

Factor

When a whole number is divided into another whole number without a remainder, it is called a factor.

Factors are either composite numbers or prime numbers (except that 0 and 1 are neither prime nor composite).

Example: The number 6 is a factor 54, because it goes into 54 evenly 9 times. In this case 9 is also a factor of 54.

Example:2 and 3 are factors of 6,because 2 × 3 = 6.

Example:3 is a factors of 9, because 3 × 3 = 9. 

Note: Prime numbers only have 2 factors: 1 and itself

Prime Factors

All factors of a number that are prime are called prime factors.

Prime Factorization

The Prime Factorization of a number is written as the product of all its prime factors.

Common Factor

A common factor is a whole number that is a factor of two or more nonzero whole numbers.

Example: 3 is a common factor of the numbers 15 and 24. Both 15 and 24 have 3 as a factor, therefore 3 is called “common” between 15 and 24.

  

Greatest Common Factor

The greatest common factor, or GCF, is the greatest factor that divides two numbers.

Different methods to find GCF

There are 3 methods for finding the GCF.

1   Method 1. Rainbow method 

Example: Find the GCF of 14, 35.

List all the factors

14: 1, 2, 7, 14

35: 1, 5, 7, 35

Common factors: 1, 7

GCF: 7

     Method 2.  Factor Tree method 

                Example: Find the GCF of 18, 24

                step 1: List all the factors using factor tree

                           18 = 2x3x3,  24 = 2x2x2x3

                step 2: Identify the common factors

                           The common factors of 18 and 24 are 2 and 3. 

                Step 3: Multiply the common factors to find the GCF.

                           GCF = 3x2 = 6

            Method 3. CAKE or ladder Method

      

Step 1:

Divide the numbers by the prime number until the numbers cannot be divided evenly

Step 2:

     Multiply all prime numbers used to divide. 

Multiple

The multiple, x, of a number, n, is a number where n is a factor of x.

Example: The number 33 (x) can be divided by 3 (n) without any remainder. So 33 is a multiple of 3 (3 * 11 = 33).

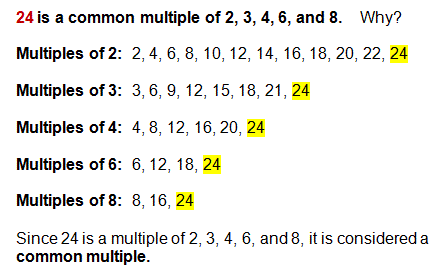
Common multiple

A common multiple is a number that is a multiple of two or more numbers. The common multiples of 3 and 4 are 12, 24, 36...

Least common multiple (LCM)

The least common multiple (LCM) is the smallest number that is a multiple of two or more numbers.

Example: The LCM of 2, 3, 4, and 6 is 12.

Different methods to find LCM

Method 1: Listing  Multiples

Find LCM of 3 and 4

Step 1:

List all multiples of 3 and 4

Step 2: 

find common multiples

Step 3:

Find least common multiple

Method 2: Using Prime Factorization

Step 1: 

Identify Common factors 

common factors for 18 and 24 are 3 and 2

step 2:

Multiply common factors with all other factors

Other factors for 18 is 3 , other factors for 24 are 2 and  2

Step 3:

Multiply the common factors with other factors

LCM = 2x3x3x2x2= 72

Method 3: ladder method

Relationship between the GCF and LCM

The product of the GCF and the LCM of two or more numbers is equal to the product of the numbers:

      GCF (a, b) · LCM (a, b) = a · b

      LCM (a,b) is read as "LCM of numbers a and b"

      GCF (a,b) is read as "GCF of numbers a and b"

Example:

    GCF (12, 16) = 4

   LCM (12, 16) = 48

   48 · 4 = 12 ·16

   192 = 192

Homework due 2/21/16

Here is next week's homework. It is Expressions and Equations  and  Worksheet 1.

Meeting of 2/14/16

 We did the Long Problem and discussed about classification of numbers.

Pythagorean Theorem

The Pythagorean Theorem states that when the squares of sides a and b on a right triangle are added up, it equals the square of side c, the hypotenuse of the triangle. 

It is more commonly known as

a² + b² = c².

(a and b are legs or sides and c is the hypotenuse)

When each of the sides of a right triangle has a whole number length, the three numbers are called a Pythagorean triple.  

Some of the Pythagorean triples are listed below:

(3,4,5) (5,12,13) (7,24,25) (8,15,17) (9,40,41) (11,60,61) (12,35,37) (13,84,85) (16,63,65) (20,21,29) (28,45,53) (33,56,65) (36,77,85) (39,80,89) (48,55,73) (65,72,97)